Loci and construction are geometric objects drawn with specific features. Drawing the locus helps us effectively identify and solve geometry problems, such as drawing diagrams to find exact distances. Loci is the set of points that follow a certain rule e.g. a circle created by points the same distance from the centre. Constructions use tools to draw shapes, bisect angles, and perpendicular distances between points and lines. These tools include a ruler, compass, and protractor.
This article is suitable for all major exam boards, including AQA and Edexcel. Quiz questions are included to test your knowledge. If you need help learning this topic, TeachTutti has experienced and DBS-checked GCSE Maths tutors to support your GCSE Maths revision.
Key principles of Loci
A locus is a set of points that meet a specific rule. For example, the locus of points at an equal distance from a single point creates a circle. They display the paths and regions in geometric problems. Common types of loci include:
- Points around a point - A circular path is created by a set distance from a central point, such as the circumference of a circle.
- Points around a line - Two parallel lines are created by points that are equal distance from a straight line, one on each side.
- Points between two points - The midpoint between two points across the length of the line. This can be found using a perpendicular bisector. All points are an equal distance from both original points.
You will often need to do scale drawings on this topic. To sketch shapes accurately, you will need a compass and ruler.
1
What is the name for the locus of points equidistant from a single point?
Geometric loci and constructions
To create accurate geometric drawings, you need a compass, ruler and protractor. Make sure to also use a pencil and rubber to allow for revisions. These are some of the common constructions for GCSE Maths:
Perpendicular bisector of a line segment
This construction finds the middle of a line segment - it keeps every point on the bisector the same distance from the endpoints of the segment. Place the compass on one endpoint and draw an arc that crosses the segment. Repeat from the other endpoint, creating two arcs that intersect above and below the line. Draw a line through these intersections to create the perpendicular bisector.
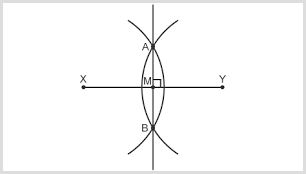
Perpendicular line from a point to a line
Place the compass on the given point and draw an arc on the line that creates two points of intersection. Draw arcs from these two intersections to create a cross-section below the line. Finally, draw a line from the point to this intersection to create a perpendicular line from a point to a line.

Angle bisector
Bisecting an angle splits it into two equal parts. Put the compass at the angle's vertex and draw an arc that crosses both rays of the angle. Put the compass on each intersection and draw two arcs that cross within the angle. Draw a line from the vertex to the intersection of these arcs to create the angle bisector.
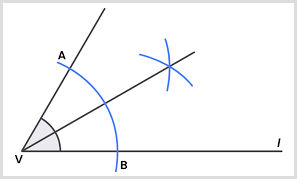
2
What appears when you create a perpendicular bisector for a line segment?
Loci and constructs in problem-solving
Loci and constructions are often used to find precise points, areas or paths. These are some of the real-life problems they can tackle:
Combined loci problems
You may need to use multiple loci to solve a problem. For example, finding points equidistant from two fixed points and within a certain distance from a line. To achieve this, combine constructions like perpendicular bisectors, parallel lines, or angle bisectors. This will display overlapping areas that satisfy each rule.
Distance from a point or line
You will often be asked to find the locus of points with a specific distance from a point. To solve these problems, use a draw to draw arcs at the required distance. This will let you place the points that fit the criteria. For instance, to find a point within 2 cm of a line, draw 2 parallel lines at this distance above and below the original line for the locus.
Bearings and directions
Bearings are used to show direction. They are always measured in degrees clockwise from North. For instance, a bearing of 045 degrees is northeast. Questions on bearings often ask you to measure or draw lines at specific angles. You will often be placed in a real-life situation, such as determining a ship's course.
3
Which construction is most appropriate when finding a region equidistant from two points?
Common mistakes
Accuracy is crucial when working with loci and constructions. These are some of the common mistakes to watch out for:
- Remove construction lines - Always leave your construction lines. They show your workings and the steps you took to solve the problem. Exams may award additional marks for correct construction lines. They confirm the accuracy of your calculations.
- Incorrect measurement of bearings - Always measure bearings clockwise starting from North. A common error is to measure bearings in the wrong direction or from an incorrect starting point. Bearings are always 3-digit numbers, even when the degree is less than 100 e.g. 90 degrees would be written 090°.
- Misjudge angles and lines - You need a steady hand and careful use of tools to get accurate angles and distances. An exam is time-sensitive, but this is one area you should never rush. For example, make sure your compass width is maintained throughout the calculation when bisecting an angle.
- Labelling diagrams - It's crucial to clearly and accurately label points, lines and angles. It makes your work easier to follow and can prevent you from losing marks (exams often give extra markings for accurate construction lines).
Final thoughts
Understanding the rules and practising will help you master loci and constructions. There are a variety of exam questions you may come across. MathsGenie has loci and construction worksheets to test your knowledge.
Make sure to use your tool carefully, keeping your compass steady and double-checking your measurements. Keep an eye on the details, such as calculating bearings clockwise from North.
If you need personalised help getting to grips with this topic, TeachTutti has a list of qualified GCSE Maths tutors to support your exam revision.
Frequently asked questions
A locus (plural loci) is a set of points that follow a specific rule, such as being the same distance from a single point. They are important in geometry as they show boundaries, paths and areas that meet certain rules. Loci allow us to draw accurate diagrams.
Construction lines show the steps taken to create a construction. They show the accuracy of your workings and often give you additional marks in examinations. Make sure to keep these lines visible in your final answer.
A perpendicular bisector divides a line segment into two equal parts - it can find points that are the same distance from two points. An angle bisector divides an angle into two equal parts - it can find points that are an equal distance from both sides of the angle.
Bearings show us direction. They are measured as three-figure angles starting from North and moving clockwise e.g. a bearing of 045° points northeast. They are a standardised system for distance, used worldwide in navigation.
You will need a ruler (straight lines and distances), compass (circles and arcs) and protractor (measure angles).
Make sure your compass is steady and set to the correct width. Double-check your calculations and avoid changing tools until you have completed the current step. Keep an eye on the specifics. For example, always start bearings from North and move clockwise.
Glossary
- Loci - A collection of points that meet a certain rule e.g. the locus of points equidistant from a single point creates a circle.
- Perpendicular bisector - A line that splits a line segment into two equal parts at a 90 degree angle, or half the length. This is used to find particular points equidistant from two endpoints.
- Bearing - A three-figure angle that shows a direction. Bearings are measured clockwise from North. They are used in navigation and expressed by numbers e.g. 180°.
- Angle bisector - A line or ray that divides an angle into two equal parts. Angle bisectors are used in constructions to locate points equidistant from the two sides of an angle.
- Compass - A drawing tool used in geometric constructions. A single or pair of compasses are often used to draw circles, arcs and points that are the same distance from a fixed point.
- Protractor - A tool used that can measure and draw angles. It is a precise method to calculate bearings or angle constructions.
- Equidistant - This means "equal distance” normally from a fixed point. They can create shapes like a line or an arc.
- Midpoint - This is exactly halfway between two other points. The midpoint of a line segment is the perpendicular bisector of the line.
- Scale drawing - An enlarged or reduced drawing proportional to the original object.
- Construction lines - The arcs and lines created in the construction process. These lines are left to show the steps taken. They are normally required in exams to show your workings.

